Review
| Site: | Clare-Gladwin RESD |
| Course: | Michigan Algebra II KHauck |
| Book: | Review |
| Printed by: | Guest user |
| Date: | Tuesday, October 21, 2025, 9:42 AM |
Description
Review
Probability is the ratio of the number of favorable outcome to the total number of outcomes. Probability is divided into two main categories: theoretical and experimental. Theoretical probability is the probability of what could happen. Experimental probability is the probability of what already happened during a trial. The following formula will be used to calculate probability:
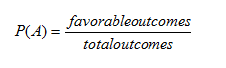
Example 1 What is the theoretical probability of getting a head when you flip a coin?
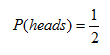
Example 2 If a coin is flipped 100 times ends on heads 57 of those times, what is the experimental probability of flipping heads?
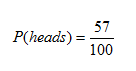
Probability Terms
Probabilities are represented by a number between 0 and 1, and can be written as a decimal, fraction or percent. A probability of 0 is called an impossible event. A probability of 1 is called a certain event. A sample space is the set of all possible outcomes of a probability experiment. It is helpful when determining the probability of an event to first organize the sample space.
Example 1 When rolling a die, what is the probability of rolling a number less than 7?
This event is certain and has a probability of 1.
Example 2 When rolling a die, what is the probability of rolling a 7?
This event is impossible and has a probability of 0.
To learn more about probability, watch the video below.
Example 3
What is the sample space of flipping three coins? Use this sample space to determine the probability of flipping three heads in a row.
The table below represents the sample space of flipping three coins.
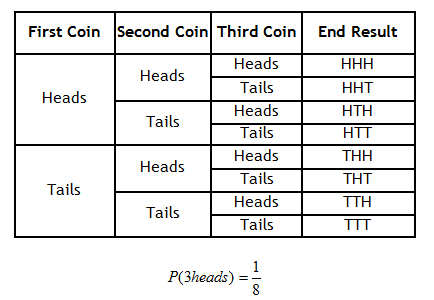
Tree Diagrams
Another way to represent a sample space is by using a tree diagram. A tree diagram is a diagram that starts at a single node, with branches emanating to additional nodes, which represent mutually exclusive decisions or events. Tree diagrams are used in strategic decision making, evaluation, or probability calculations.
Example 1
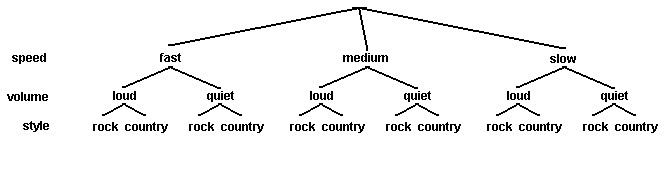
You are the proud inventor of the SongWriter 2000â„¢. The user sets the song speed ("fast," "medium," or "slow"), the volume ("loud" or "quiet"), and the style ("rock" or "country"). Then, the SongWriter automatically writes a song to match. Make a tree diagram to determine how many possible settings there are.
|
|
Starting at the top of the tree and following all the way down, you end up with one particular kind of song such as, "fast loud country song." There are 12 different song types in all. The number of outcomes is found by multiplying the number of settings for each knob:
3 × 2 × 2 = 12 and is an example of the counting principle.
To learn more about the counting principle, watch the following video.
Example 2
Suppose the machine has a "Randomize" setting that randomly chooses the speed, volume, and style. What is the probability that you will end up with a loud rock song that is not slow?
Step 1. Count the total number of favorable results.
There are two results that match the criterion:
"fast-loud-rock" and "medium-loud-rock"
Step 2. Count the total number of results.
There are 12 total results possible.
Step 3. Use the probability formula.
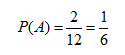
This means that non-slow, loud rock songs should happen 1 out of every 6 times. In other words, there is a 17% probability of any given song matching this description.
Video Lesson
To learn more about theoretical probability, select the following link:
Finding Theoretical ProbabilityGuided Practice
To solidify your understanding of probability, visit the following link to Holt, Rinehart, and Winston Homework Help Online. It provides examples, video tutorials, and interactive practice with answers available. The Practice and Problem Solving section has two parts. The first part offers practice with a complete video explanation for the type of problem with just a click of the video icon. The second part offers practice with the solution for each problem only a click of the light bulb away.
Guided PracticePractice
Counting Problems Worksheet*Note: If Google Docs displays, “Sorry, we were unable to retrieve the document for viewing,†refresh your browser.
Answer Key
Counting Problems Answer Key*Note: If Google Docs displays, “Sorry, we were unable to retrieve the document for viewing,†refresh your browser.
Independent & Dependent
Independent events are defined as outcomes that are not affected by other outcomes. In other words, the flip of the penny does not affect the flip of the nickel, and vice versa. Dependent events are outcomes that are affected by other outcomes. In other words, pulling a blue marble from a hat and leaving it out will affect the probability of the remaining marbles on the next draw.
To learn more about independent and dependent events, view the video:
To learn more about independent and dependent events, select the following links:
Conditional Probability
A conditional probability contains a restriction that may limit the sample space for a given event. For example, if the sample space is all shirt colors in your class, then the probability of red would consider the entire class. However, the probability of a red shirt given it is on a girl, would limit the sample space to only the girls in the class.
This type of probability is denoted by P(red | girl).
To read more about conditional probability, select the following link:
To learn more about conditional events, view the video:
Practice
Investigating Probability Worksheet*Note: If Google Docs displays, “Sorry, we were unable to retrieve the document for viewing,†refresh your browser.
Answer Key
Investigating Probability Answer Key*Note: If Google Docs displays, “Sorry, we were unable to retrieve the document for viewing,†refresh your browser.
Sources
Sources used in this book:CliffsNotes.com. Conditional Probability. 28 Jul 2010 <http://www.cliffsnotes.com/study_guide/topicArticleId-25951,articleId-25916.html>.
CliffsNotes.com. Dependent Events. 28 Jul 2010
<http://www.cliffsnotes.com/study_guide/topicArticleId-25951,articleId-25912.html>.
CliffsNotes.com. Probability of Simple Events. 28 Jul 2010
<http://www.cliffsnotes.com/study_guide/topicArticleId-25951,articleId-25911.html>.
Embracing Mathematics, Assessment & Technology in High Schools; a Michigan Mathematics & Science Partnership Grant Project
Felder, K. (2009, April 15). Probability Concepts -- Probability. Retrieved from the Connexions Web site: http://cnx.org/content/m19073/1.2/
Glencoe, McGraw-Hill, "Theoretical and Experimental Probability." http://www.glencoe.com/sec/math/studytools/cgi-bin/msgQuiz.php4?isbn=0-07-829633-1&chapter=9&lesson=6&headerFile=4&state=na (accessed 7/27/2010).
Holt, Rinehart & Winston, "Probability and Statistics."http://my.hrw.com/math06_07/nsmedia/homework_help/alg2/alg2_ch11_02_homeworkhelp.html (accessed 7/27/2010).