Measures of Center
| Site: | Clare-Gladwin RESD |
| Course: | Michigan Algebra II KHauck |
| Book: | Measures of Center |
| Printed by: | Guest user |
| Date: | Wednesday, October 22, 2025, 3:18 AM |
Description
Measures of Center
A measure of center is a value at the center or middle of a data set. Graphically, the center can be viewed as the "balance point" of the display. Algebraically, the most common ways to find the center are with the mean, median, or mode. Mean is the average or sum of all data points divided by the number of points. The notation used for mean is the greek letter mu, ![]() . Median is the middle value. In order to find the median, put the points in increasing order and find the middle value. If there is an even number of data points, find the average between the two middle points. The notation used for median is a capital letter m, M. Mode is the most often occurring number.
. Median is the middle value. In order to find the median, put the points in increasing order and find the middle value. If there is an even number of data points, find the average between the two middle points. The notation used for median is a capital letter m, M. Mode is the most often occurring number.
Example
Find the mean, median, and mode of the following set of data:
2, 5, 6, 4, 8, 9, 8, 7, 5, 2, 2, 3, 10, 1, 4, 1, 10, 5, 2
Step 1. To find the mean, add the numbers together and divide by the number of data items.
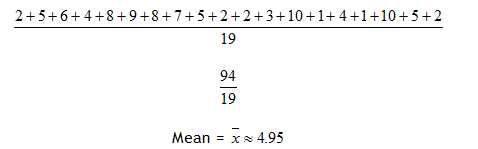
Step 2. To find the median, put the numbers in increasing order and then find the middle.
1, 1, 2, 2, 2, 2, 3, 4, 4, 5, 5, 5, 6, 7, 8, 8, 9, 10, 10
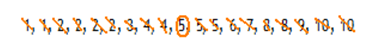
Median = 5
Step 3. To find the mode, determine which number appears most often.
Two appears more than any other number.
Mode = 2
Best Measure
Different measures of center are appropriate for different data sets. When choosing the best measure for the data, always try to find all three to have as much information as possible. Mode is the only possible measure when dealing with categorical variables and should also be chosen when asked for the most popular response. Mean is the measure of center that is affected by outliers. A large outlier will skew the mean high and a small outlier will skew the mean low. When an outlier is present, the median is the best measure of center to represent the data. Mean is the best measure of center when there is no outlier.
Example 1
Which is the best measure of center for real estate values in a county where there is both inland and lake front property?
Since the price of lake front property is considerably higher than inland property, it would represent an outlier. Median is the best representation of this data.
Example 2
Which is the best measure of center for a company where 100 employees earn $5000 per year and the CEO makes $2,000,000 per year?
The CEO would be considered an outlier and skew the mean high. When representing the employees, the mode would be the best choice.
Video Lessons
To learn how to find mean, median, and mode select one of the following links:
Finding Measures of Central Tendency
Finding Mean, Median, Mode & Range of a Data Set
To learn how to choose the best measure of center, select the following link:
Guided Practice
To solidify your understanding of mean, median, and mode, visit the following link to Holt, Rinehart, and Winston Homework Help Online. It provides examples, video tutorials, and interactive practice with answers available. The Practice and Problem Solving section has two parts. The first part offers practice with a complete video explanation for the type of problem with just a click of the video icon. The second part offers practice with the solution for each problem only a click of the light bulb away.
Practice
*Note: If Google Docs displays, "Sorry, we were unable to retrieve the document for viewing," refresh your browser.
Answer Key
*Note: If Google Docs displays, "Sorry, we were unable to retrieve the document for viewing," refresh your browser.
Sources
Sources used in this book:Embracing Mathematics, Assessment & Technology in High Schools; a Michigan Mathematics & Science Partnership Grant Project
Holt, Rinehart & Winston, "Choosing a Measure of Central Tendency."Holt, Rinehart & Winston, "Data Analysis and Probability."
Holt, Rinehart & Winston, "Finding Mean, Median, Mode and Range."
Holt, Rinehart & Winston, "Finding Measures of Central Tendency."
Schultzkie, Lisa. "Measures of Central Tendency."