Print bookPrint book

Hyperbolas

Site: Clare-Gladwin RESD
Course: Michigan Algebra II KHauck
Book: Hyperbolas
Printed by: Guest user
Date: Tuesday, November 26, 2024, 12:24 AM

Description

Hyperbolas

Table of contents

Hyperbolas

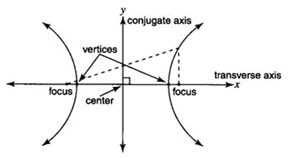
A hyperbola is the set of all points in a plane such that the absolute value of the difference of the distances between two fixed points stays constant. The two given points are the foci of the hyperbola, and the midpoint of the segment joining the foci is the center of the hyperbola. The hyperbola looks like two opposing "U-shaped" curves.
hyperbolas


A hyperbola has two axes of symmetry. The axis along the direction the hyperbola opens is called the transverse axis. The conjugate axis passes through the center of the hyperbola and is perpendicular to the transverse axis. The points of intersection of the hyperbola and the transverse axis are called the vertices of the hyperbola.


Asymptotes

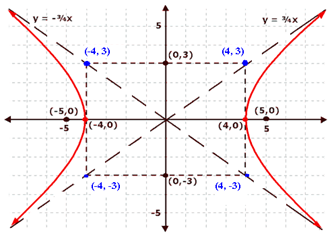
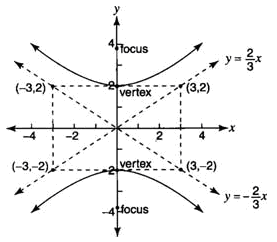
As points on a hyperbola get farther from its center, they get closer and closer to two lines called asymptote lines. The asymptote lines are used as guidelines in sketching the graph of a hyperbola. To find equations of the asymptotes, use point-slope form of an equation of a line or form a rectangle by using the points (?a, b), (-a, -b), (a, b), and (a, - b) and draw its diagonals as extended lines. These diagonals are the asymptotes. See the graph of:

Asymptotes1

Asymptotes2




Transverse Axis

x-axis
A hyperbola centered at (0, 0) whose transverse axis is along the x-axis has the following equation in standard form.

TransverseX1Vertices: (a, 0) and (-a, 0)

Foci: (c, 0) and (-c, 0), where c2 = a2 + b2

Equation of asymptote lines: TransverseX2
y-axis
A hyperbola centered at (0, 0) whose transverse axis is along the y-axis has the following equation in standard form.

TransverseY1
Vertices: (0, a) and (0, -a)

Foci: (0, c) and (0, -c), where c2 = a2 + b2

Equation of asymptote lines: TransverseY2


In general, when a hyperbola is written in standard form, the transverse axis is along, or parallel to, the axis of the variable that is not being subtracted.

Hyperbolas vs. Ellipse

Similarities between Hyperbolas and Ellipses:
  • The formula is identical, except for the replacement of a "+" with a "-".
  • The definition of a is very similar. In a horizontal ellipse, you move horizontally a units from the center to the edges of the ellipse (This defines the major axis). In a horizontal hyperbola, you move horizontally a units from the center to the vertices of the hyperbola (This defines the transverse axis). b defines a different, perpendicular axis.
  • The definition of c is identical: the distance from center to focus.
Differences between Hyperbolas and Ellipses:
  • The biggest difference is that for an ellipse, a is always the biggest of the three variables; for a hyperbola, c is always the biggest. This should be evident from looking at the drawings (the foci are inside an ellipse, outside a hyperbola). However, this difference leads to several other key distinctions.
  • For ellipses, c2= a2-b2. For hyperbolas, c2=a2+b2.
  • For ellipses, you tell whether it is horizontal or vertical by looking at which denominator is greater, since a must always be bigger than b. For hyperbolas, you tell whether it is horizontal or vertical by looking at which variable has a positive sign, the x2 or the y2. The relative sizes of a and b do not distinguish horizontal from vertical.

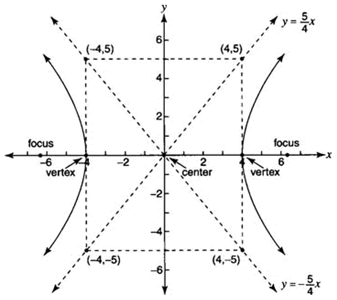
Example 1

Graph the following hyperbola. Find its center, vertices, foci, and the equations of its asymptote lines.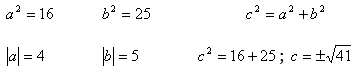
HyperbolasEx1-1

Step 1. Identify the center, vertices, foci, and the equations of asymptotes.

This is a hyperbola with center at (0, 0), and its transverse axis is along the x-axis.

HyperbolasEx1-2

Vertices: (-4, 0), (4, 0)

Foci: (?41, 0), (-?41, 0)

Equations of asymptote lines: HyperbolasEx1-3
Step 2 . Graph the conic.
HyperbolasEx1-4

Example 2

Graph the following hyperbola. Find its center, vertices, foci, and the equations of its asymptote lines.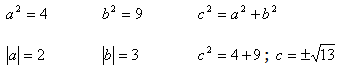
HyperbolasEx2-1
Step 1 . Identify the center, vertices, foci, and the equations of asymptotes.

This is a hyperbola with center at (0, 0), and its transverse axis is along the y-axis.

HyperbolasEx2-2

Vertices: (0, 2), (0, -2)

Foci: (0, ?13), (0, -?13)

Equations of asymptote lines: HyperbolasEx2-3

Step 2 . Graph the conic.
HyperbolasEx2-4

Center (h, k)

A hyperbola centered at (h, k) will have the following standard equations:

  • If the transverse axis is horizontal, then

HyperbolasCenter1-1

Vertices: (h+a, k), (h-a, k)

Foci: (h+c, k), (h-c, k), where c2 = a2 + b2

Equations of asymptote lines: HyperbolasCenter1-2

  • If the transverse axis is vertical, then

HyperbolasCenter2-3

Vertices: (h, k+a) and (h, k-a)

Foci: (h, k+c) and (h, k-c), where c2 = a2 + b2

Equations of asymptote lines: HyperbolasCenter1-4

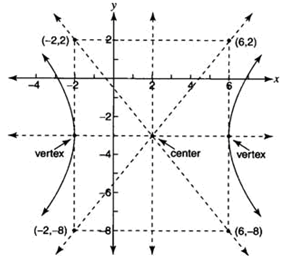
Example 3

Graph the following equation.
HyperbolasEx3-1
Step 1 . Identify the conic section.

This is a hyperbola because the x and y terms are both squared and the y term is subtracted from the x term, creating a horizontal transverse axis.

Step 2 . Identify the center, vertices, and the corners of the rectangle.

Center: (2, ?3)

Vertices: (2 + 4, -3) and (2 - 4, -3) or (6, -3) and (-2, -3)

Coordinates of the corners of the rectangle:
  • (2 + 4, -3 + 5) = (6, 2)
  • (2 - 4, -3 + 5) = (-2, 2)
  • (2 + 4, -3 - 5) = (6, -8)
  • (2 - 4, -3 - 5) = (-2, -8)
Step 3 . Graph the conic.

HyperbolasEx3-2

Symmetry

Hyperbolas have both reflectional and rotational symmetry. The reflectional symmetry of every hyperbola is a vertical line through the center and a horizontal line through the center. The rotational symmetry of every hyperbola is 180 degrees about the center point.

Example State the symmetry for the hyperbola modeled by the equation:

HyperbolaRevised1

Step 1. State the center.

The center of the hyperbola is: (1, -5)

Step 2. State the reflectional symmetry.

This hyperbola has lines of symmetry at x = 1 and y = -5.

Step 3. State the rotational symmetry.

This hyperbola has 180 degree rotational symmetry about (1, -5).

Nonstandard Form

If the equation is not given in standard form, it will need to be converted to standard form by completing the square. To identify an equation as a hyperbola, it will contain an x2 and y2 term where only one has a negative coefficient as in the example below.

Example 4 Convert the following equation into standard form.
HyperbolasEx4-1

Step 1 . Identify the conic section.

This is a hyperbola because the x and y terms are both squared, and only one term has a negative coefficient, the y term.

Step 2 . Write equation in standard form.

(2x2+4x) + (-1y2+4y) = 4; Group the x terms, group the y terms

2(x2+2x) + -1(y2-4y) = 4; Factor the leading coefficient from each group

2(x2+2x+1) + -1(y2-4y+4) = 4 + (2?1) + (-1?4); Complete the square for x and y

2(x+1)2 + -1(y-2)2 = 2; Simplify

HyperbolasEx4-2 ; Divide each side of the equation by 2 and simplify.
 

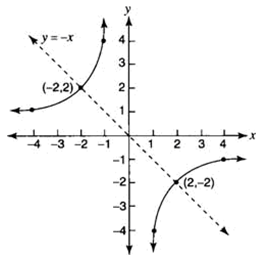
Diagonal Hyperbola

The equation xy = 16 also represents a hyperbola. This hyperbola has its center at (0, 0), and its transverse axis is the line y = x. The asymptotes are the x- and y-axes. Its vertices are at (?16, ?16) and (-?16, -?16). The graph is shown below.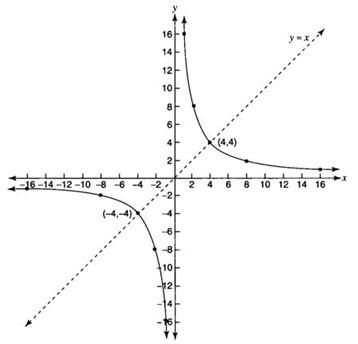
DiagonalHyperbola1

Example 5

Graph the following equation.
HyperbolasEx5-1

Step 1 . Identify the properties of the conic.

This hyperbola has its center at (0, 0). The transverse axis is the line y = -x. The asymptotes are the x- and y-axes. The vertices are at (?4, -?4) and (-?4, ?4) or (2, -2) and (-2, 2).

Step 2 . Graph the conic.

HyperbolasEx5-2
 

Video Lessons

To learn how to graph hyperbolas, select the following link:

Graph Hyperbolas

To learn how to write the equation of a hyperbola, select the following link:

Equation of a Hyperbola

Guided Practice

To solidify your understanding of graphing and writing equations of hyperbolas, visit the following link to Holt, Rinehart, and Winston Homework Help Online. It provides examples, video tutorials and interactive practice with answers available. The Practice and Problem Solving section has two parts. The first part offers practice with a complete video explanation for the type of problem with just a click of the video icon. The second part offers practice with the solution for each problem only a click of the light bulb away.

Guided Practice

Practice

Hyperbola Worksheet

*Note: If Google Docs displays, "Sorry, we were unable to retrieve the document for viewing," refresh your browser.
 

Video Lessons

To see more examples on hyperbolas, view the videos below.

Graphing Hyperbola Center Origin


Hyperbola Video Two

 

Optional Note Taking Guide


Answer Key

Answer Key for Hyperbola Worksheet

 
*Note: If Google Docs displays, "Sorry, we were unable to retrieve the document for viewing," refresh your browser.
 

Sources

Sources used in this book:

CliffsNotes.com. Hyperbola. 27 Jul 2010 <http://www.cliffsnotes.com/study_guide/topicArticleId-38949,articleId- 38944.html>.

Green, Larry. "Finding the Equation of the Ellipse and the Hyperbola." http://www.ltcconline.net/greenl/java/IntermedCollegeAlgebra/ConicGraphT oEquation/ConicGraphToEquation.html (accessed 7/27/2010).

Green , Larry. "Graphing Hyperbolas Applet." http://www.ltcconline.net/greenl/java/IntermedCollegeAlgebra/Hyperbola/H yperbola.htm (accessed 7/27/2010).

Green, Larry. "Graphing the Ellipse and the Hyperbola." http://www.ltcconline.net/greenl/java/IntermedCollegeAlgebra/EllipseHyperb ola/EllipseHyperbola.html (accessed 7/27/2010).

Holt, Rinehart & Winston, "Conic Sections ." http://my.hrw.com/math06_07/nsmedia/homework_help/alg2/alg2_ch10_04_ homeworkhelp.html (accessed 7/27/2010).

Kenny Felder, "Conic Concepts -- Circles," Connexions, March 22, 2010, http://cnx.org/content/m18245/1.3/.