Simplifying Rationals

Site:	Clare-Gladwin RESD
Course:	Michigan Algebra II KHauck
Book:	Simplifying Rationals

Printed by:	Guest user
Date:	Saturday, November 23, 2024, 6:04 PM

Description

Simplify

Undefined Expressions
Lowest Terms
- Example 2
- Example 3
Common Mistakes
Video Lesson
Practice
- Answer Key
Sources

Undefined Expressions

A rational expression , where n(x) and d(x) are polynomial expressions is defined when . Since division by 0 is undefined, the expression(s) in the denominator can not equal zero. Values that make an expression in the denominator equal to zero are excluded values.

Example Find excluded value(s) if they exist.

$frac2$

Step 1. Factor the expression in the denominator.

$frac3$

Step 2. Set each factor in the denominator equal to zero.

x + 1 = 0 or x + 2 = 0

Step 3. Solve each equation.

x = -1 or x = -2

Therefore, -1 and -2 are the excluded values for x.

Lowest Terms

A rational expression in lowest terms has no common factors in the numerator and denominator. To write an expression in lowest terms or simplest form, factor the numerator and denominator and cancel common factors.

Example 1 Reduce the rational expression to lowest terms:

$frac1$

Step 1. Factor the numerator and denominator completely.

$frac2$

Step 2. Cancel the common factor of 2x.

$frac3$

Step 3. Rewrite.

$frac4$ (Answer)

Example 2

Reduce the rational expression to simplest form:

$frac4$

Step 1. Factor the numerator and denominator completely.

$frac5$

Step 2. Cancel the common factor of (x-1).

$frac6$

Step 3. Rewrite.

$frac6$ (Answer)

Example 3

Reduce the rational expression to lowest terms:

Step 1. Factor the numerator and denominator completely.

$frac8$

Step 2. Cancel the common factor of (x-2) .

crossout

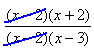
Step 3. Rewrite.

(Answer)

Common Mistakes

When reducing expressions, you are only allowed to cancel common factors but NOT common terms. For example, in the expression $frac1$ the (x-3) factor can be canceled because $frac2$ . Therefore, $frac3$ .

However, don't make the mistake of canceling out common terms in the numerator and denominator. For instance, in the expression cancelling the x² terms will result in $frac2$ . However, substituting x = 2 in the expression results in $frac3$ , which is not the same answer as above. When we cancel out terms that are part of a sum or a difference we are violating the order of operations. $frac4$ Try this with numbers: when the 9s are cancelled first. This method produces a different solution; therefore it is an incorrect method!