Graphs
| Site: | Clare-Gladwin RESD |
| Course: | Michigan Algebra I |
| Book: | Graphs |
| Printed by: | Guest user |
| Date: | Wednesday, January 15, 2025, 3:46 PM |
Description
Graphs
From A Graph
The steps to model a polynomial function from a graph are very similar to the steps used when given a table. First, determine the zeros of the function by looking at the graph. Second, turn each zero into a factor of the function. Finally, determine the factored form of the polynomial.Example 1 Determine the function that models the graph below:
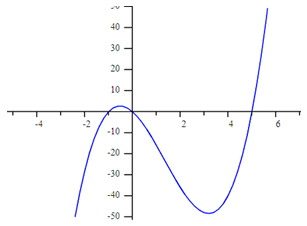
Step 1. Determine the zeros of the function.
(-1, 0), (0, 0), (5, 0)
Step 2. Turn each zero into a factor of the function.
Since x = -1, then (x + 1) is a factor of the polynomial.
Since x = 0, then (x) is a factor of the polynomial.
Since x = 5, then ( x - 5) is a factor of the polynomial.
Example 1 Continued
y = a (x + 1)(x)(x - 5); point (4, -40)
-40 = a (4 + 1)(4)(4 - 5)
-40 = a (5)(4)(-1)
-40 = a (-20)
2 = a
Example 2
Determine the function that models the graph below: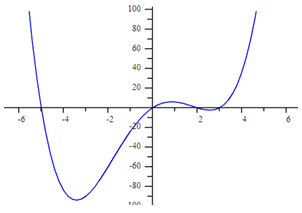
Step 1. Determine the zeros of the function.
(-5, 0), (0, 0), (2, 0), (3, 0)
Step 2. Turn each zero into a factor of the function.
Since x = -5, then (x + 5) is a factor of the polynomial.
Since x = 0, then (x) is a factor of the polynomial.
Since x = 2, then (x - 2) is a factor of the polynomial.
Since x = 3, then (x - 3) is a factor of the polynomial.
Step 3. Determine the factored form of the polynomial by using another point from the graph. Substitute the point in for (x, y) and solve for the value of a.
y = a (x + 5)(x)(x - 2)(x - 3); point (-2, -60)
-60 = a (-2 + 5)(-2)(-2 - 2)(-2 - 3)
-60 = a (3)(-2)(-4)(-5)
-60 = a (-120)
.5 = a
Practice
Polynomials Equations WorksheetAnswer Key
Polynomials Equations Answer KeySource
Embracing Mathematics, Assessment & Technology in High Schools; A Michigan Mathematics & Science Partnership Grant Project